Shape of the Distribution, Normality

An important aspect of the description of a variable is the shape of its distribution, which tells you the frequency of values from different ranges of the variable.

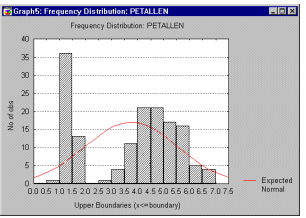
Typically, a researcher is interested in how well the distribution can be approximated by the normal distribution. Simple descriptive statistics can provide some information relevant to this issue. For example, if the skewness (which measures the deviation of the distribution from symmetry) is clearly different from 0, then that distribution is asymmetrical, while normal distributions are perfectly symmetrical. If the kurtosis (which measures peakedness of the distribution) is clearly different from 0, then the distribution is either flatter or more peaked than normal; the kurtosis of the normal distribution is 0.

More precise information can be obtained by performing one of the tests of normality to determine the probability that the sample came from a normally distributed population of observations. For example, the so-called Kolmogorov-Smirnov test, or the Shapiro-Wilk W test. However, none of these tests can entirely substitute for a visual examination of the data using a histogram (i.e., a graph that shows the frequency distribution of a variable). To easily generate a histogram from a spreadsheet, right-click on a cell within the desired variable and select Histogram from the Graphs of Input Data shortcut menu.

The graph enables you to evaluate the normality of the empirical distribution because it also shows the normal curve superimposed over the histogram. It also allows you to examine various aspects of the distribution qualitatively. For example, the distribution could be bimodal (have 2 peaks). This might suggest that the sample is not homogeneous but possibly its elements came from two different populations, each more or less normally distributed. In such cases, in order to understand the nature of the variable in question, you should look for a way to quantitatively identify the two sub-samples.