Analyzing Invariance Properties
Some important recent papers (Browne & Shapiro, 1989, 1991; Dijkstra, 1990) have investigated invariance properties of covariance structures. This topic is important for several reasons. First, some of the statistics calculated (i.e., the G1 and G2 coefficients) depend on a certain scale invariance property. Second, some analyses discussed in the preceding section depend on invariance properties of the covariance structure. Finally, and perhaps most important, to fully understand the relationship between data and model, it is crucial to understand what aspects of the model are affected by rescalings of the data, and what aspects (if any) are unaffected.
Shapiro and Browne (1989) established a number of key invariance properties for covariance structures. Their paper concerned situations where a model fitting a covariance matrix S of a random vector X would continue to fit if the manifest variables in x were linearly transformed, i.e.,
(145)
Shapiro and Browne (1989) studied how covariance structures remained invariant when S is allowed to be transformed by A matrices of various types. They used the following definition. Consider a multiplicative group G of nonsingular p x p matrices. That is, if AÎG and BÎG, then
AB-1ÎG. Under certain side conditions met by the groups under consideration, G constitutes a Lie group with matrix multiplication as the group operation. Associated with G is a corresponding Lie group G* of transformations defined on the set of symmetric positive definite matrices by S ®
ASA' . A covariance structure is a symmetric matrix valued function S(q) which relates a parameter vector
q from a subset
of Âq to S.
- Definition
- A covariance structure S(q) is said to be invariant under the group G* if for every
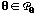and AÎG there exists a
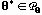such that S(q*) = AS(q*)A'.
This means that for any AÎG, the set of positive definite matrices corresponding to the given model remains invariant under the transformation S ® ASA'.
Browne and Shapiro (1989) studied several types of A matrices corresponding to different kinds of invariance.
These included two kinds of invariance which are of particular interest to SEPATH users (see Types of Invariance).