Example 17: A Test for Compound Symmetry
A test for compound symmetry (i.e., equal variances and correlations for all pairs of variables) of the covariance matrix is sometimes performed in the context of repeated measures analysis of variance (see, e.g., Winer, 1971, pages 596-598). This hypothesis states that the covariance matrix has equal diagonal elements, and equal off-diagonal elements. The Winer.sta data file contains the pooled covariance matrix analyzed by Winer.
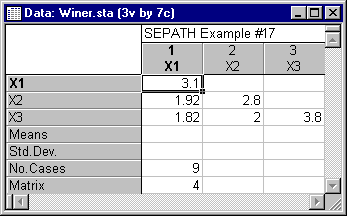
Because the matrix was obtained by pooling two samples of size 5, it would have the same distribution (assuming both populations have the same covariance matrix) as a covariance matrix based on a single sample of size 9. Hence, that is the sample size recorded in the data file. We test that the covariance matrix has the form

Commands for testing the hypothesis are in the file Winer.cmd (also in the /Examples/Datasets/SEPATH directory of STATISTICA). You can run this model by clicking the Open model button on the Advanced tab of the Structural Equation Modeling dialog, choosing this file, and clicking the OK (Run model) button.
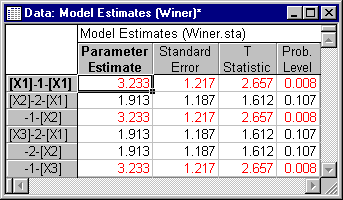
On the Quick tab of the Structural Equation Modeling Results dialog, click the Model summary button. Note that the maximum likelihood estimates under the null hypothesis correspond to the results on page 598 of the Winer text.
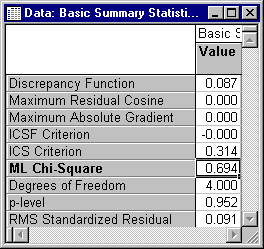
If you click the Basic summary statistics button, you will find that the Chi-square statistic, with 4 degrees of freedom, is only .694. This value is not significant. In fact, the probability level is suspiciously high, as is sometimes seen with artificial data in ANOVA textbooks! This fact, coupled with the high values for the fit coefficients, suggests that the population matrix deviates only trivially from the hypothesized structure.